【高校数学】三角比の対称式（交代式）の問題【テスト・入試によく出るパターンを網羅！】

三角比の対称式（交代式）で、テストや大学入試によく出るパターンの問題を網羅したい！

三角比の対称式を、基礎からはじめて 入試の基本レベルまで徐々に学んでいきたい！

こういった要望をお持ちの人に向けて、この記事を書きました。

このページを最後まで読めば、三角比の対称式（交代式）の問題で、テストや大学入試でよく出るパターンが網羅 できます。

できるだけ図を使いながら、丁寧に分かりやすく解説してあります。

実際に手を動かして問題を解きながら一緒に学んでいきましょう！

対称式（交代式）について

「対称式（交代式）」について、おさらいしたい人はこちら。

関連記事

【高校数学】対称式（交代式）の解き方をわかりやすく解説【基本定理はこれだけ覚えよう】

対称式・交代式の解き方がよくわからない そもそも対称式って何？ 対称式のわかりやすい解説が聞きたい！ 今回はこういった疑問・要望にお答えします。 対称式（交代式）の問題は、解法が完全にパターン化されています（やり方が決ま[…]

三角比について

「三角比の相互関係」の公式について、おさらいしたい人はこちら。

関連記事

【三角比の相互関係】公式3つの覚え方・使い方・証明【簡単＆超シンプルにわかりやすく解説】

「三角比の相互関係」の3つの公式が覚えられない！ 簡単に暗記したり、証明する方法を知りたい！ 「三角比の相互関係」をいつ使うのか、タイミングや問題を教えてほしい！ こういった要望に応えます。   「三角比[…]

「三角比の相互関係」の3公式の中でも、特に多く使うのが

このページの問題でもガンガン使うので、しっかり頭に入れておきましょう！

三角比の対称式（交代式）Lv.1【基本問題】

まずは「三角比の対称式（交代式）」の基本問題から。

和 → 積 を求める問題

対称式はとりあえず「和の式を2乗する」と覚えておきましょう。

【解答】を見る

【解答】

$ $　$ \displaystyle { \sin \theta + \cos \theta = {1 \over 4} }$

の両辺を2乗して

$ $　$ \displaystyle { ( \sin \theta + \cos \theta )^2 = \left( {1 \over 4} \right)^2 } $

$ $　∴ $ \displaystyle { \color{red} { \sin^2 \theta } + 2 \sin \theta \cos \theta + \color{red} { \cos^2 \theta } = {1 \over 16} } $

ここに $ \color{red} {\sin^2 \theta + \cos^2 \theta} = 1 $ を代入して

$ $　$ \displaystyle { \color{red} {1} + 2 \sin \theta \cos \theta = { 1 \over 16} } $

$ $　∴ $ \displaystyle { 2 \sin \theta \cos \theta = −{15 \over 16} } $

$ $　∴ $ \displaystyle { \sin \theta \cos \theta = −{15 \over 32} } $

差 → 積 を求める問題

今回は「差の式」が与えられているので、やはり 2乗 してみます。

【解答】を見る

【解答】

$ $　$ \displaystyle { \sin \theta − \cos \theta = −{ \sqrt{2} \over 3} }$

の両辺を2乗して

$ $　$ \displaystyle { ( \sin \theta − \cos \theta )^2 = \left( −{ \sqrt{2} \over 3} \right)^2 } $

$ $　∴ $ \displaystyle { \color{red} { \sin^2 \theta } − 2 \sin \theta \cos \theta + \color{red} { \cos^2 \theta } = {2 \over 9} } $

ここに $ \color{red} {\sin^2 \theta + \cos^2 \theta} = 1 $ を代入して

$ $　$ \displaystyle { \color{red} {1} − 2 \sin \theta \cos \theta = {2 \over 9} } $

$ $　∴ $ \displaystyle { −2 \sin \theta \cos \theta = −{7 \over 9} } $

$ $　∴ $ \displaystyle { \sin \theta \cos \theta = {7 \over 18} } $

三角比の対称式（交代式）Lv.2【応用問題1】

次に「三角比の対称式（交代式）」の応用問題をやってみましょう！

ちょっと問題数が多いですが、対称式の頻出パターンが網羅できます！

(1) $\sin \theta \cos \theta$

やり方は先ほどの基本問題と同じですね。

【解答】を見る

【解答】

$ $　$ \displaystyle { \sin \theta + \cos \theta = {1 \over 2} }$ ・・・①

の両辺を2乗して

$ $　$ \displaystyle { ( \sin \theta + \cos \theta )^2 = \left( {1 \over 2} \right)^2 } $

$ $　∴ $ \displaystyle { \color{red} { \sin^2 \theta } + 2 \sin \theta \cos \theta + \color{red} { \cos^2 \theta } = {1 \over 4} } $

ここに $ \color{red} {\sin^2 \theta + \cos^2 \theta} = 1 $ を代入して

$ $　$ \displaystyle { \color{red} {1} + 2 \sin \theta \cos \theta = {1 \over 4} } $

$ $　∴ $ \displaystyle { 2 \sin \theta \cos \theta = −{3 \over 4} } $

$ $　∴ $ \displaystyle { \sin \theta \cos \theta = −{3 \over 8} } $ ・・・②

(2) $\sin^3 \theta + \cos^3 \theta$

3乗の形を作るために「展開の公式」を利用します。

【解答】 を見る

【解答】

①の両辺を3乗して

$ $　$ \left( \sin \theta + \cos \theta \right)^3 = \left( \displaystyle { 1 \over 2 } \right)^3 $

$ $　∴ $ \sin^3 \theta + 3 \sin^2 \theta \cos \theta + 3 \sin \theta \cos^2 \theta + \cos^3 \theta = \displaystyle { 1 \over 8 } $

$ $　∴ $ \sin^3 \theta + \cos^3 \theta + 3 \sin^2 \theta \cos \theta + 3 \sin \theta \cos^2 \theta = \displaystyle { 1 \over 8 } $

$ $　∴ $ \sin^3 \theta + \cos^3 \theta + 3 \color{green} { \sin \theta \cos \theta } \enspace ( \color{red} { \sin \theta + \cos \theta } ) = \displaystyle { 1 \over 8 } $

ここに

$ \begin{cases}
① \color{red} { \sin \theta + \cos \theta } = \displaystyle { 1 \over 2 } \\
\\
② \color{green} { \sin \theta \cos \theta } = − \displaystyle { 3 \over 8 } \\
\end{cases}$

を代入して

$ $　$ \displaystyle { \sin^3 \theta + \cos^3 \theta + 3 \cdot \left( \color{green} {−  { 3 \over 8 } } \right) \cdot \color{red} { 1 \over 2 } = { 1 \over 8 } } $

$ $　∴ $ \displaystyle { \sin^3 \theta + \cos^3 \theta − { 9 \over 16 } = { 1 \over 8 } } $

$ $　∴ $ \displaystyle { \sin^3 \theta + \cos^3 \theta = { 1 \over 8 } + { 9 \over 16 } } $

$ $　　　　　　　　 $ \displaystyle { = { 11 \over 16 } } $

別解として「因数分解の公式」を使ってもできます。

【別解】を見る

【別解】

$ \sin^3 \theta + \cos^3 \theta $

$ = ( \color{red} { \sin \theta + \cos \theta } ) ( \sin^2 \theta − \color{green} { \sin \theta \cos \theta  } + \cos^2 \theta ) $

ここに

$ \begin{cases}
\sin^2 \theta + \cos^2 \theta = 1 \\
\\
① \color{red} { \sin \theta + \cos \theta } = \displaystyle { 1 \over 2 } \\
\\
② \color{green} { \sin \theta \cos \theta } = − \displaystyle { 3 \over 8 } \\
\end{cases}$

を代入して

（与式）$\displaystyle { = \color{red} { 1 \over 2 } \left\{ 1 − \left( \color{green} { −  { 3 \over 8 } } \right) \right\} } $

$ $　　　　$ \displaystyle { = { 1 \over 2 } \cdot { 11 \over 8 } } $

$ $　　　　$ \displaystyle { = { 11 \over 16 } } $

(3) $\displaystyle { \tan \theta + {1 \over \tan \theta} } $

$\tan \theta $ を消去 → 分数を通分 すると、対称式が代入できそうな形になります。

【解答・解説】を見る

【解答】

$\displaystyle { \tan \theta + { 1 \over \tan \theta } }$

$\displaystyle { = { \sin \theta \over \cos \theta } + { \cos \theta \over \sin \theta } }$　$\displaystyle { \left( \tan \theta = {\sin \theta \over \cos \theta} を代入 \right) }$

$\displaystyle { = { \color{red} { \sin^2 \theta + \cos^2 \theta } \over \color{green} { \sin \theta \cos \theta } } }$　・・・（注1）

 

ここに

$ \begin{cases}
\color{red} { \sin^2 \theta + \cos^2 \theta } = 1 \\
\\
② \color{green} { \sin \theta \cos \theta } = − \displaystyle { 3 \over 8 } \\
\end{cases}$

を代入して

（与式）$\displaystyle { = { \color{red} { 1 } \over \displaystyle \color{green} {−{ 3 \over 8 } } } } $

$ $　　　　$\displaystyle { = { 1 × 8 \over \displaystyle {−{ 3 \over 8 }× 8 } } } $ ・・・（注2）

$ $　　　　$\displaystyle { = − { 8 \over 3 } } $

（注1）ここの通分の流れは、例えば

$ $　$\displaystyle { { 4 \over 5 } + { 5 \over 4 } } $

$ $　$\displaystyle { = { 4^2 \over 5 \cdot 4 } + { 5^2 \over 4 \cdot 5 } } $

$ $　$\displaystyle { = { 4^2 + 5^2 \over 5 \cdot 4 } } $

と同様に考えると

$ $　$\displaystyle { { \sin \theta \over \cos \theta } + { \cos \theta \over \sin \theta } }$

$ $　$\displaystyle { = { \sin^2 \theta \over \cos \theta \sin \theta } + { \cos^2 \theta \over \sin \theta \cos \theta } }$

$ $　$\displaystyle { = { \sin^2 \theta + \cos^2 \theta \over \sin \theta \cos \theta } }$

 

（注2）分数の中に分数が入っているときは、「分母 $\left( \displaystyle {−{ 3 \over 8 } } \right)$ と分子 $\left( 1 \right)$ に同じ数 $\left( 8 \right)$ をかける」と考えるといいです。

あるいは、「割り算（分子 ÷ 分母）」とみなして

$ $　$\displaystyle { { 1 \over \displaystyle {−{ 3 \over 8 } } } } $

$ $　$\displaystyle { = 1 ÷ \left(−{ 3 \over 8 } \right) } $

$ $　$\displaystyle { = 1 × \left(−{ 8 \over 3 } \right) } $

と計算してもOK。

(4) $\sin \theta − \cos \theta$

$\sin \theta − \cos \theta$ の値をいきなり求めることはできませんが、

とりあえず 2乗した形  $ ( \sin \theta − \cos \theta )^2 $ なら分かりそうですよね？

【解答・解説】を見る

【解答】

$ ( \sin \theta − \cos \theta )^2 $

$ = \color{red} { \sin^2 \theta } − 2 \color{green} { \sin \theta \cos \theta } + \color{red} { \cos^2 \theta } $

$ = \color{red} { 1 } − 2 \cdot \left( \color{green} { − \displaystyle { 3 \over 8 } } \right)  $　$ \left( \color{red} { \sin^2 \theta + \cos^2 \theta } = 1 、 ② \color{green} { \sin \theta \cos \theta } = − \displaystyle { 3 \over 8 } を代入 \right) $

$ \displaystyle { = 1 + { 3 \over 4 } } $

$ \displaystyle { = { 7 \over 4 } } $

 

ここで、$0^\circ ≦\theta≦180^\circ$ より $\sin \theta ≧ 0$ ・・・（注1）

また、$\sin \theta \cos \theta ＜0$ より $\sin \theta$、$\cos \theta$ は異符号。

これらを合わせて考えると $\cos \theta ＜0$ ・・・（注2）

∴ $− \cos \theta ＞0$

 

「$\sin \theta ≧ 0$ かつ $− \cos \theta ＞0$」より

$\sin \theta − \cos \theta ＞0 $・・・（注3）

 

ゆえに

$ \displaystyle { \sin \theta − \cos \theta = \sqrt{ 7 \over 4 } }$

$ $　　　　　$ \displaystyle { = { \sqrt{ 7 } \over 2 } } $・・・③

（注1）「$0^\circ ≦\theta≦180^\circ$」より、単位円をかくと

なので、$\sin \theta ≧ 0$（$0$以上）とわかります。

 

（注2）$\sin \theta \cos \theta ＜0$（かけてマイナス）ということは

$\sin \theta$、$\cos \theta$ のうち一方は「+」、もう一方は「−」（異符号）になります。

上記の(注1)より $\sin \theta ≧ 0$（$\color{red}{0}$以上）なので、もう一方の $\cos \theta$ は「−」と分かります。

 

（注3）$\sin \theta − \cos \theta$ は、

「$\sin \theta ≧ 0$（$\color{red}{0}$以上）」と「$− \cos \theta ＞0$（+）」を足した数なので

$\sin \theta − \cos \theta＞0$（+）になります。

別解として、$\color{red} { \cos \theta }$ を消去 して「$ \sin \theta $ の2次方程式」を解くやり方もあります。

【別解】を見る

【別解】

$ \displaystyle { \sin \theta + \cos \theta = {1 \over 2} }$ より

$ $　$ \displaystyle { \color{red} { \cos \theta } = {1 \over 2} − \sin \theta }$

これを $ \sin^2 \theta + \color{red} { \cos } ^2 \color{red} { \theta } = 1 $ に代入して

$ $　$ \displaystyle { \sin^2 \theta + \left( \color{red} { {1 \over 2} − \sin \theta } \right)^2 = 1 } $

$ $　∴ $ \displaystyle { \sin^2 \theta + {1 \over 4} −\sin \theta + \sin^2 \theta  = 1 } $

$ $　∴ $ \displaystyle { 2 \sin^2 \theta −\sin \theta − {3 \over 4} = 0 } $

両辺に $4$ をかけて

$ $　$ 8 \sin^2 \theta −4 \sin \theta − 3 = 0 $

これを $\sin \theta$ についての2次方程式と見て、解を求めると

$ $　$ \displaystyle { \sin \theta = { 2 \pm \sqrt{ 4 −8 \cdot (−3) } \over 8 } }$ ・・・（注1）

$ $　　　$ \displaystyle { = { 2 \pm \sqrt{ 28 } \over 8 } }$

$ $　　　$ \displaystyle { = { 2 \pm 2 \sqrt{ 7 } \over 8 } }$

$ $　　　$ \displaystyle { = { 1 \pm \sqrt{ 7 } \over 4 } }$

 

ここで、$0^\circ ≦\theta≦180^\circ$ より　$ 0 ≦\sin \theta≦1 $

$ $　∴ $ \displaystyle { \color{green} {\sin \theta} = { 1 + \sqrt{ 7 } \over 4 } }$ ・・・（注2）

 

よって

$ $　$ \cos \theta = \displaystyle {1 \over 2} − \color{green} {\sin \theta} $

$ $　　$ = \displaystyle {1 \over 2} − \color{green} { 1 + \sqrt{ 7 } \over 4 } $

$ $　　$ = \displaystyle { 1 − \sqrt{ 7 } \over 4 } $

 

ゆえに

$ $　$\sin \theta −\cos \theta$

$ $　$ \displaystyle { = { 1 + \sqrt{ 7 } \over 4 } − { 1 − \sqrt{ 7 } \over 4 } } $

$ $　$ \displaystyle { = { 2 \sqrt{ 7 } \over 4 } } $

$ $　$ \displaystyle { = { \sqrt{ 7 } \over 2 } } $

（注1）$ \displaystyle { 8x^2 −4x −3 = 0 }$ の解き方について

ふつうの解の公式を使ってしまうと

$ $　$ \displaystyle { x = { 4 \pm \sqrt{ 4^2 −4 \cdot 8 \cdot (−3) } \over 2 \cdot 8 } }$

となって計算がすこし大変ですね。

そこで

$x$ の2次方程式  $ax^2 + bx + c = 0$

において $b$ が偶数のとき、$b = 2b’$ とすると

$ \displaystyle { x = { −b’ \pm \sqrt{ b’^2 − ac } \over a } }$

これを利用すると

$ $　$ \displaystyle { x = { 2 \pm \sqrt{ 2^2 − 8 \cdot (−3) } \over 8 } }$

と、ちょっとだけ楽ができます。

 

（注2）ここで

「$\displaystyle { \sin \theta = { 1 + \sqrt{ 7 } \over 4 } } $ は、$ 0 ≦\sin \theta≦1 $ の範囲にホントに入ってるの？$\sqrt{ 7 }$ の値なんて覚えてないよ〜」

と思ったキミへ・・・

もし $\sqrt{ 7 }$ の値を知らなくても

$ $　$2^2 ＜ 7＜ 3^2$ より　$2 ＜\sqrt{ 7 }＜ 3$

というのは分かりますよね？

ここから $\displaystyle { 1 + \sqrt{ 7 } \over 4 }$ の形に少しずつ近づけていきます。

 

$ $　$2 ＜\sqrt{ 7 }＜ 3$

辺々に $1$ を足して

$ $　$3 ＜1 + \sqrt{ 7 }＜ 4$

辺々を $4$ で割って

$ $　$\displaystyle { {3 \over 4}＜ { 1 + \sqrt{ 7 } \over 4 }＜{4 \over 4} }$

$ $　∴ $\displaystyle { {3 \over 4}＜\sin \theta＜1 }$

となり、$ 0 ≦\sin \theta≦1 $ を満たしていることが分かりました。

この変形のしかたは、これ以外にも 2次関数など色んな場面で必要となります。

バッチリ覚えておきましょう！

(5) $\sin \theta$

「$\sin \theta$ と $\cos \theta$ の和・差」が分かっているので、そこから $\sin \theta$ の値を求められます。

【解答】を見る

【解答】

$ \begin{cases}
① \sin \theta + \cos \theta = \displaystyle { 1 \over 2 } \\
\\
③ \sin \theta − \cos \theta  = \displaystyle { \sqrt{ 7 } \over 2 } \\
\end{cases}$

の辺々を足して

$ $　$ \displaystyle { ( \sin \theta + \require{cancel} \bcancel{ \cos \theta } ) + ( \sin \theta − \bcancel{ \cos \theta } ) =  { 1 \over 2 } + { \sqrt{ 7 } \over 2 } } $

$ $　∴ $ \displaystyle { 2 \sin \theta =  { 1 + \sqrt{ 7 } \over 2 } } $

$ $　∴ $ \displaystyle { \sin \theta = { 1 \over 2 } \cdot { 1 + \sqrt{ 7 } \over 2 } } $

$ $　　　　 $ \displaystyle { =  { 1 + \sqrt{ 7 } \over 4 } } $

(6) $\displaystyle { \tan \theta − { 1 \over \tan \theta } }$

$\tan \theta $ を消去 → 分数を通分 → 因数分解 すると、対称式が代入できそうな形になります。

【解答】を見る

【解答】

$\displaystyle { \tan \theta − { 1 \over \tan \theta } }$

$\displaystyle { = { \sin \theta \over \cos \theta } − { \cos \theta \over \sin \theta } }$　$\displaystyle { \left( \tan \theta = {\sin \theta \over \cos \theta} を代入 \right) }$

$\displaystyle { = { \sin^2 \theta − \cos^2 \theta \over \sin \theta \cos \theta } }$

$\displaystyle { = { ( \color{red} { \sin \theta + \cos \theta } ) ( \color{blue} { \sin \theta − \cos \theta } ) \over \color{green} { \sin \theta \cos \theta } } }$

 

ここに

$ \begin{cases}
① \color{red} { \sin \theta + \cos \theta } = \displaystyle { 1 \over 2 } \\
\\
② \color{green} { \sin \theta \cos \theta } = − \displaystyle { 3 \over 8 } \\
\\
③ \color{blue} { \sin \theta − \cos \theta } = \displaystyle { \sqrt{ 7 } \over 2 } \\
\end{cases}$

を代入して

（与式）$\displaystyle { = { \displaystyle { \color{red} { 1 \over 2 } \cdot \color{blue} { \sqrt{ 7 } \over 2 } } \over \displaystyle { \color{green} {− { 3 \over 8 } } } } } $

$ $　　　　$\displaystyle { = { \enspace  \displaystyle { \sqrt{7} \over 4 } \enspace \over \displaystyle {−{ 3 \over 8 } } } } $

$ $　　　　$\displaystyle { = { \displaystyle { { \sqrt{7} \over 4 }× 8 } \over \displaystyle {−{ 3 \over 8 }× 8 } } } $

$ $　　　　$\displaystyle { = − { 2 \sqrt{7}  \over 3 } } $

(7) $ \tan \theta $

一見難しそうに見えますが、

(3)と(6) の結果から「$\tan \theta$ と $\displaystyle { 1 \over \tan \theta} $ の和・差」が分かるので、そこから $\tan \theta$ の値を求められます。

【解答】を見る

【解答】

(3)、(6) より

$ \begin{cases}
\displaystyle { \tan \theta + { 1 \over \tan \theta } = − { 8 \over 3 } } \\
\\
\displaystyle { \tan \theta − { 1 \over \tan \theta } = − { 2 \sqrt{7}  \over 3 } }\\
\end{cases}$

辺々を足して

$ $　$ \displaystyle { \left( \tan \theta + \require{cancel} \bcancel{ 1 \over \tan \theta } \right) + \left( \tan \theta − \bcancel { 1 \over \tan \theta } \right) =  − { 8 \over 3 } − { 2 \sqrt{7}  \over 3 } } $

$ $　∴ $ \displaystyle { 2 \tan \theta =  − { 8 + 2 \sqrt{7}  \over 3 } } $

$ $　∴ $ \displaystyle { \tan \theta =  − { 8 + 2 \sqrt{7}  \over 3 } \cdot {1 \over 2} } $

$ $　　　　 $ \displaystyle { =  − { 4 + \sqrt{7}  \over 3 } } $

三角比の対称式（交代式）Lv.3【応用問題2】

もうひとつ「三角比の対称式（交代式）」の応用問題です。

場合分け が必要なパターンを見ておきましょう。

(1) $\sin \theta \cos \theta$

【解答】を見る

【解答】

$ $　$ \displaystyle { \sin \theta + \cos \theta = { \sqrt{5} \over 2} } $ ・・・①

の両辺を2乗して

$ $　$ \displaystyle { \left( \sin \theta + \cos \theta \right)^2 = \left( { \sqrt{5} \over 2} \right)^2 } $

$ $　∴ $ \displaystyle { \color{red} { \sin^2 \theta } + 2 \sin \theta \cos \theta + \color{red} {\cos^2 \theta} = {5 \over 4} } $

ここに $ \color{red} {\sin^2 \theta + \cos^2 \theta} = 1$ を代入して

$ $　$ \displaystyle { \color{red} { 1 } + 2 \sin \theta \cos \theta  = {5 \over 4} } $

$ $　∴ $ \displaystyle { 2 \sin \theta \cos \theta  = {1 \over 4} } $

$ $　∴ $ \displaystyle { \sin \theta \cos \theta  = {1 \over 8} } $ ・・・②

(2) $\sin \theta − \cos \theta$

$( \sin \theta − \cos \theta )^2 $ を考えるのは先ほどと同じですが、

$\sin \theta − \cos \theta$ の符号が決まらないので場合分けがいりますね。

【解答・解説】を見る

【解答】

$ ( \sin \theta − \cos \theta )^2 $

$ = \color{red} { \sin^2 \theta } − 2 \color{green} { \sin \theta \cos \theta } + \color{red} { \cos^2 \theta } $

$ = \color{red} { 1 } − 2 \cdot \color{green} { \displaystyle { 1 \over 8 } }  $　$ \left( \color{red} { \sin^2 \theta + \cos^2 \theta } = 1 、 ② \color{green} { \sin \theta \cos \theta } = \displaystyle { 1 \over 8 } を代入 \right) $

$ \displaystyle { = 1 −{ 1 \over 4 } } $

$ \displaystyle { = { 3 \over 4 } } $

 

ここで、$0^\circ ≦\theta≦180^\circ$ より $\sin \theta ≧ 0$ ・・・（注1）

また、$\sin \theta \cos \theta ＞0$ より $\sin \theta$、$\cos \theta$ は同符号。

これらを合わせて考えると

$ $　$\sin \theta ＞0$、$\cos \theta ＞0$ ・・・（注2）

$ $　∴ $0^\circ ＜\theta＜90^\circ$

 

[1] $\sin \theta − \cos \theta ＞0$ すなわち $\sin \theta ＞ \cos \theta $

$ $　すなわち $45^\circ ＜\theta＜90^\circ$ のとき

$ $　　$\displaystyle { \sin \theta − \cos \theta = \sqrt{ 3 \over 4 } } $

$ $　　　　　　 $\displaystyle { = { \sqrt{ 3 } \over 2 } } $

 

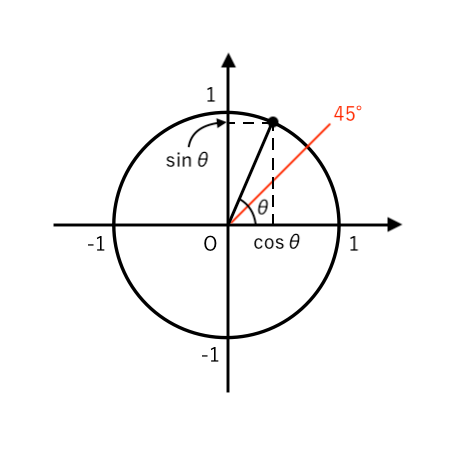
[2] $\sin \theta − \cos \theta = 0$ すなわち $\sin \theta = \cos \theta $

$ $　すなわち $ \theta = 45^\circ $ のとき

$ $　　$\displaystyle { \sin \theta − \cos \theta = 0 } $

 

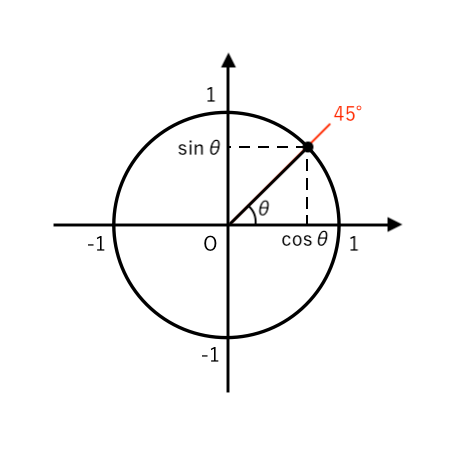
[3] $\sin \theta − \cos \theta ＜0$ すなわち $\sin \theta ＜ \cos \theta $

$ $　すなわち $0^\circ ＜\theta＜45^\circ$ のとき

$ $　　$\displaystyle { \sin \theta − \cos \theta = − \sqrt{ 3 \over 4 } } $

$ $　　　　　　　 $\displaystyle { = − { \sqrt{ 3 } \over 2 } } $

 

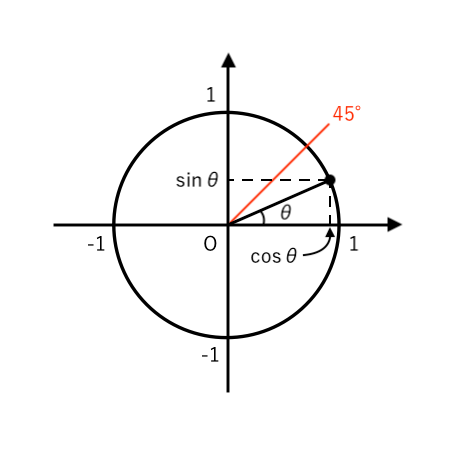
以上より

$ $　$\sin \theta − \cos \theta =
\begin{cases}
\displaystyle { − { \sqrt{ 3 } \over 2 } } \enspace ( 0^\circ ＜\theta＜45^\circ ) \\
\\
\enspace 0 \enspace \enspace \enspace ( \theta = 45^\circ ) \\
\\
\displaystyle { { \sqrt{ 3 } \over 2 } } \enspace \enspace ( 45^\circ ＜\theta＜90^\circ ) \\
\end{cases}$

（注1）「$0^\circ ≦\theta≦180^\circ$」より、単位円をかくと

なので、$\sin \theta ≧ 0$（$0$以上）とわかります。

 

（注2）$\sin \theta \cos \theta ＞0$（かけてプラス）ということは

$\sin \theta$、$\cos \theta$ の符号は「+・+」または「−・−」（同符号）になります。

上記の(注1)より $\sin \theta ≧ 0$（$\color{red}{0}$以上）なので、もう一方の $\cos \theta$ も「+」と分かります。

三角比の対称式（交代式）Lv.4【入試問題】

最後に「三角比の対称式（交代式）」の 入試問題 に挑戦です。

ここまでの総復習も兼ねて練習してみましょう。

これが解けたら「三角比の対称式」は怖いもの無しです！ がんばりましょう！

(1) $\sin \theta \cos \theta$

【解答】を見る

【解答】

$ $　$ \displaystyle { \sin \theta + \cos \theta = {1 \over 3} }$ ・・・①

の両辺を2乗して

$ $　$ \displaystyle { ( \sin \theta + \cos \theta )^2 = \left( {1 \over 3} \right)^2 } $

$ $　∴ $ \displaystyle { \color{red} { \sin^2 \theta } + 2 \sin \theta \cos \theta + \color{red} { \cos^2 \theta } = {1 \over 9} } $

ここに $ \color{red} {\sin^2 \theta + \cos^2 \theta} = 1 $ を代入して

$ $　$ \displaystyle { \color{red} {1} + 2 \sin \theta \cos \theta = { 1 \over 9 } } $

$ $　∴ $ \displaystyle { 2 \sin \theta \cos \theta = − { 8 \over 9 } } $

$ $　∴ $ \displaystyle { \sin \theta \cos \theta = − { 4 \over 9 } } $ ・・・②

(2) $\sin^3 \theta + \cos^3 \theta$

【解答】を見る

【解答】

①の両辺を3乗して

$ $　$ \left( \sin \theta + \cos \theta \right)^3 = \left( \displaystyle { 1 \over 3 } \right)^3 $

$ $　∴ $ \sin^3 \theta + 3 \sin^2 \theta \cos \theta + 3 \sin \theta \cos^2 \theta + \cos^3 \theta = \displaystyle { 1 \over 27 } $

$ $　∴ $ \sin^3 \theta + \cos^3 \theta + 3 \sin^2 \theta \cos \theta + 3 \sin \theta \cos^2 \theta = \displaystyle { 1 \over 27 } $

$ $　∴ $ \sin^3 \theta + \cos^3 \theta + 3 \color{green} { \sin \theta \cos \theta } \enspace ( \color{red} { \sin \theta + \cos \theta } ) = \displaystyle { 1 \over 27 } $

ここに

$ \begin{cases}
① \color{red} { \sin \theta + \cos \theta } = \displaystyle { 1 \over 3 } \\
\\
② \color{green} { \sin \theta \cos \theta } = − \displaystyle { 4 \over 9 } \\
\end{cases}$

を代入して

$ $　$ \displaystyle { \sin^3 \theta + \cos^3 \theta + 3 \cdot \left( \color{green} {−  { 4 \over 9 } } \right) \cdot \color{red} { 1 \over 3 } = { 1 \over 27 } } $

$ $　∴ $ \displaystyle { \sin^3 \theta + \cos^3 \theta − { 4 \over 9 } = { 1 \over 27 } } $

$ $　∴ $ \displaystyle { \sin^3 \theta + \cos^3 \theta = { 1 \over 27 } + { 4 \over 9 } } $

$ $　　　　　　　　 $ \displaystyle { = { 13 \over 27 } } $ ・・・③

(3) $\sin^4 \theta + \cos^4 \theta$

4乗 ＝ (2乗)$^2$ と考えると指針が見えてきます。

【解答】を見る

【解答】

$ $　$\sin^2 \theta + \cos^2 \theta = 1$

の両辺を2乗して

$ $　$ \left( \sin^2 \theta + \cos^2 \theta \right)^2 = 1^2$

$ $　∴ $ \sin^4 \theta + 2 \sin^2 \theta \cos^2 \theta + \cos^4 \theta = 1$

$ $　∴ $ \sin^4 \theta + 2 ( \color{green} { \sin \theta \cos \theta } )^2 + \cos^4 \theta  = 1$

$ $　∴ $ \displaystyle { \sin^4 \theta + 2 \left( \color{green} {− { 4 \over 9 } } \right)^2 + \cos^4 \theta  = 1 } $ （②を代入）

$ $　∴ $ \displaystyle { \sin^4 \theta + { 32 \over 81 } + \cos^4 \theta  = 1 } $

$ $　∴ $ \displaystyle { \sin^4 \theta + \cos^4 \theta  = 1 − { 32 \over 81 } } $

$ $　　　　　　　　 $ \displaystyle { = { 49 \over 81 } } $

(4) $\sin^4 \theta − \cos^4 \theta$

まずは因数分解してみましょう。すると、やるべきことが分かってきます。

【解答】を見る

【解答】

$\sin^4 \theta − \cos^4 \theta$

$ = \left(\sin^2 \theta \right)^2 − \left(\cos^2 \theta \right)^2 $

$ = \left( \sin^2 \theta + \cos^2 \theta \right) \left( \sin^2 \theta − \cos^2 \theta \right) $

$ = \left( \sin^2 \theta + \cos^2 \theta \right) ( \sin \theta + \cos \theta ) ( \sin \theta − \cos \theta ) $

 

$ \sin \theta − \cos \theta $ の値を求める。

$ ( \sin \theta − \cos \theta )^2 $

$ = \color{red} { \sin^2 \theta } − 2 \color{green} { \sin \theta \cos \theta } + \color{red} { \cos^2 \theta } $

$ = \color{red} { 1 } − 2 \cdot \left( \color{green} { − \displaystyle { 4 \over 9 } } \right)  $　$ \left( \color{red} { \sin^2 \theta + \cos^2 \theta } = 1 、 \color{green} {②} を代入 \right) $

$ \displaystyle { = 1 + { 8 \over 9 } } $

$ \displaystyle { = { 17 \over 9 } } $

 

ここで、$0^\circ ＜\theta＜180^\circ$ より $\sin \theta ＞ 0$

また、$\sin \theta \cos \theta ＜0$ より $\sin \theta$、$\cos \theta$ は異符号。

これらを合わせて考えると $\cos \theta ＜0$

∴ $− \cos \theta ＞0$

 

「$\sin \theta ＞ 0$ かつ $− \cos \theta ＞0$」より

$ $　$\sin \theta − \cos \theta ＞0 $

 

ゆえに

$ \displaystyle { \sin \theta − \cos \theta = \sqrt{ 17 \over 9 } }$

$ $　　　　　$ \displaystyle { = { \sqrt{ 17 } \over 3 } } $・・・④

 

よって

$\sin^4 \theta − \cos^4 \theta$

$ = \left( \sin^2 \theta + \cos^2 \theta \right) ( \color{red} { \sin \theta + \cos \theta } ) ( \color{blue} { \sin \theta − \cos \theta } ) $

$ \displaystyle { = 1 \cdot \color{red} { 1 \over 3 } \cdot \color{blue} { \sqrt{ 17 } \over 3 } } $　$ \left( \sin^2 \theta + \cos^2 \theta = 1 、 \color{red} {①} 、\color{blue} {④} を代入 \right) $

$ \displaystyle { = { \sqrt{ 17 } \over 9 } } $

(5) $\sin^5 \theta + \cos^5 \theta$

5乗ってどうやって作るの？ と考えると、

5乗 ＝ 2乗 × 3乗 で作れそうですね。

$ $　$ x^5 + y^5 = \left( x^2 + y^2 \right) \left( x^3 + y^3 \right) − x^3 y^2 −x^2 y^3 $

$ $　　　　$ = \left( x^2 + y^2 \right) \left( x^3 + y^3 \right) −x^2 y^2 \left( x + y \right)$

これは「対称式の公式」として覚えておきましょう。

【解答】を見る

【解答】

$ \sin^5 \theta + \cos^5 \theta$

$= \left( \sin^2 \theta + \cos^2 \theta \right) \left( \sin^3 \theta + \cos^3 \theta \right)$

$ $　　$−\sin^2 \theta \cos^2 \theta ( \sin \theta + \cos \theta ) $

$ = \left( \sin^2 \theta + \cos^2 \theta \right) \left( \sin^3 \theta + \cos^3 \theta \right)$

$ $　　$− ( \sin \theta \cos \theta )^2 ( \sin \theta + \cos \theta ) $

$\displaystyle { = 1 \cdot { 13 \over 27 } − \left( − { 4 \over 9 } \right)^2 \cdot { 1 \over 3 } } $　$ \left( \sin^2 \theta + \cos^2 \theta = 1 、①、②、③ を代入 \right) $

$\displaystyle { = { 13 \over 27 } − { 16 \over 243 } } $

$\displaystyle { = { 101 \over 243 } } $

別解として、(3)で「4乗の和」が求めてあるので、5乗 ＝ 4乗 × 1乗 と考えると

$ $　$ x^5 + y^5 = \left( x^4 + y^4 \right) \left( x + y \right) − x^4 y −x y^4 $

$ $　　　　$ = \left( x^4 + y^4 \right) \left( x + y \right) −x y \left( x^3 + y^3 \right)$

この考え方は、以下のように一般化されています。

この公式は、数学B「数列（漸化式）」の問題でも出てきます。詳しく知りたい人は「3項間の漸化式（ぜんかしき）」などで調べてみてください。

今度はこれを使って解いてみましょう！

【別解】を見る

【別解】

$ \sin^5 \theta + \cos^5 \theta$

$= \left( \sin^4 \theta + \cos^4 \theta \right) \left( \sin \theta + \cos \theta \right)$

$ $　　$−\sin \theta \cos \theta \left( \sin^3 \theta + \cos^3 \theta \right) $

$\displaystyle { = { 49 \over 81 } \cdot { 1 \over 3 } − \left( − { 4 \over 9 } \right) \cdot { 13 \over 27 } } $

$\displaystyle { = { 49 \over 243 } + { 52 \over 243 } } $

$\displaystyle { = { 101 \over 243 } } $

最後に

お疲れ様でした！！

これで「三角比の対称式（交代式）」の分野で、テスト・大学入試によく出るパターン をひと通りやり終えました。

対称式の考え方は、三角比 以外の分野でも使う場面が多いので、しっかりマスターしておきましょう！

質問・要望があれば気軽にコメントください👍